The Self-Similar Coast

Below is a brief introduction to J. R. Carpenter's The Pleasure of the Coast: A Hydrographic Novel (2019).
Read The Pleasure of the Coast for yourself online here.
Then, consider registering to join a free, live, open group discussion with J. R. Carpenter – scheduled for this Saturday, January 16th at 12 PM EST.
In The Fractal Geometry of Nature, mathematician Benoit B. Mandelbrot returns to an earlier question of his, "How Long Is the Coast of Britain?" To start, he suggests,
The result is most peculiar: coastline length turns out to be an elusive notion that slips between the fingers of one who wants to grasp it. All measurement methods ultimately lead to the conclusion that the typical coastline's length is very large and so ill determined that it is best considered infinite.
The ability to measure and compare the length of different coasts, however, has practical value. Going through various methods of approximating length, he leads up to his point that "Coastlines are fractal patterns." In measuring, one must add in the lengths of constant irregularities, and then the irregularities within those smaller samples of coastline, and so on infinitely.

The point, ultimately, is locating the mathematical pattern in nature.
"the infinite coast" is the first section of J. R. Carpenter's 2019 The Pleasure of the Coast: A Hydrographic Novel. Below a wide (5,252 pixel) image extending past both ends of the browser window, a horizontal scroll bar rests centrally, inviting the reader to scroll either right or left. A selection of six such images will rotate in endlessly, removing any bound to one's scrolling.
The images are sketches of coastline with some notes, and in the second section, "the technical coast," smaller details are highlighted within a similarly endless scroll. Now, however, it is not only the images which rotate, but text as well, "a circular memory: the impossibility of living outside the infinite coast."
Reworking text from Roland Barthes' The Pleasue of the Text, Beautemps-Beupré's Introduction to the Practice of Nautical Surveying and the Construction of Sea-Charts, and Jean Giraudoux's Suzanne et le Pacific, Carpenter reflects on the coast: as experienced, as surveyed, as approached in language – and the translations within and between those categories.
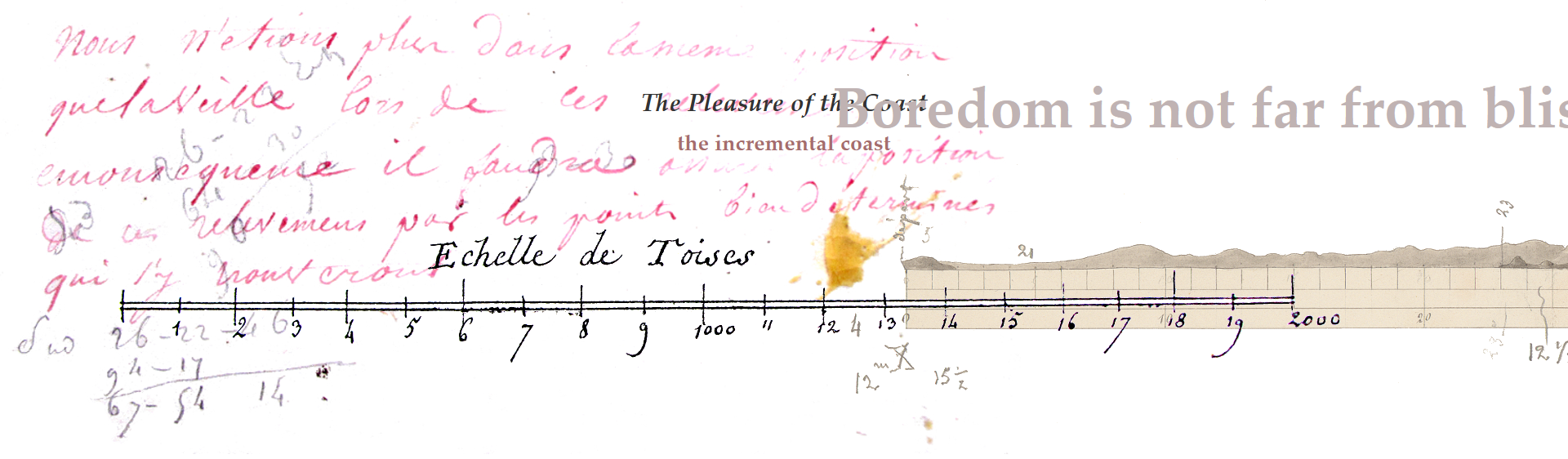
Like Mandelbrot imagining taking an increasingly infinite number of yardsticks to measure the coast, the slippage between modes of understanding and between languages (French and English) leaves us no end. In crafting this digital text, Carpenter repeats this further through the endless scrolling, the endless cycling of text, and the loops between the five pages of text and image.
Fractal geometry is about self-similarity. The whole has the shape of a part, and the part gives us the shape of the whole, and this repeats endlessly. For our narrative purposes, we therefore do not need an end, but can instead trace out patterns to better understand both the part and the whole.
This looser form of navigating – what Carpenter in her about page for the work calls perusing (which is also, she suggests, in a way, pursuing) – also has no definite end. Despite that lack of narrative closure, when the purpose is exploring the personal pleasure of the coast – which is the also here the pleasure of the text – we can simply enjoy the dive as long as we can manage, drawing out more through each iteration.
Further Reading:
"Digital Nature" – On J. R. Carpenter's This is a Picture of Wind: A Weather Poem for Phones, a 2018 work which is currently updating with new content on Twitter.
"Hearing Islands, Islands That Hear" – On J. R. Carpenter's Etheric Ocean.
"Navigational Text" – on J. R. Carpenter's ...and by islands I mean paragraphs. (Coming Friday, January 15.)
As noted at the top, J. R. Carpenter will be speaking on Zoom this Saturday. All are welcome.